The arbitrage-free law (or Kirchhoff’s voltage law)
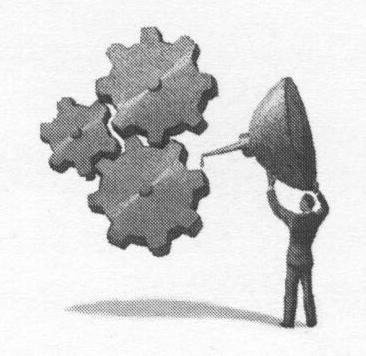
Recently I emailed a friend to complain when his organization used this 3 gear image as their logo. What was my complaint? Read on.
The basic idea of arbitrage is to “get something for nothing” by trading commodities or currencies around some circle ending up with more than one started with. The simplest example would be to have a commodity which can be bought and sold at two different prices. Then a clear profit is obtained by the arbitrage operation of buying low and selling high.
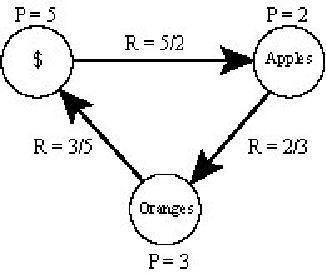
The circular pattern is that money is transformed into so many units of the commodity at the low price. Say $1 dollar buys 4 apples so each apple is priced at 25¢. Then these units of the commodity are transformed back into money at the high price, say at 50¢ per apple so the 4 apples would sell for $2. The arbitrage profit is the additional money received back over the money originally paid out to purchase the commodity, in this case $2 – $1 = $1. We assume that no transactions costs are involved with the exchanges.
Such an example of arbitrage profit could not be sustained for long since everyone would want to make the circular trades and get something for nothing. Those who were willing to sell apples at 25¢ would raise their prices and those who were willing to buy apples for 50¢ would offer less until an equilibrium price was reached somewhere between 25¢ and 50¢, say at 40¢. Then we would have a situation called “arbitrage-free” where circular trades would just break even (always assuming no transactions costs).
When profitable arbitrage was possible, then apples in effect had two prices. When the market is arbitrage-free, then the “law of one price” holds. Then and only then can prices be assigned to the commodities being traded so that all exchange rates are just price ratios. For instance, when the one price of an apple at 40¢ prevailed, then $1 could be exchanged for 2.5 = 5/2 apples and 1 apple could be exchanged for 2/5 of a dollar.
Another way to characterize the arbitrage-free situation is that if you multiply exchange rates around a circle, then they have to multiple to 1 as in (5/2) x (2/5) = 1 which simply says that if you start with $1 and make the circular exchange, then you have to end with $1. In a market with many commodities (and no transaction costs), then the market is arbitrage-free if for any possible circular exchange (e.g., dollars for apples for oranges for dollars), the product of all the exchange rates has to be 1.
(5/2) x (2/3) x (3/5) = 1
Each exchange ratio is the ratio (tail/head) of the prices of the goods. If in going around a circle, an arrow is traversed in the opposite direction, then one uses the reciprocal of its exchange rate. The basic mathematical result about being arbitrage-free is:
there exists prices for the goods so that the exchange rates are the price ratios if and only if the product of the exchange rates around any circle is 1.
In an early use of mathematical methods in economics, this law was observed by Cournot in 1838 [Cournot, Augustin. 1897 (orig. 1838). Mathematical Principles of the Theory of Wealth Trans. Nathaniel Bloom. New York: Macmillan]. But the better-known version of the mathematical result was the additive version. To translate from the multiplicative case to the additive case, replace 1 with 0, replace the ratio of two numbers with the difference of the two numbers, and replace multiplying around the circle with adding around the circle. Then the law becomes Kirchhoff’s voltage law which could be formulated as:
there exists potentials at the nodes of an electrical network so that the voltage on the wire between two nodes is the potential difference if and only if the sum of the voltages around any circle is 0.
Although the mathematical result is usually attributed to Kirchhoff [Kirchhoff, G. 1847. “Über die Auflosung der Gleichungen, auf welche man dei der Untersuchung der linearen Verteilung galvanischer Strome gefuhrt wird.” Annalen der Physik und Chemie 72: 497-508], Cournot had precedence by a few years.
The arbitrage-free law in social networks
The arbitrage-free law (or KVL if one prefers) has applications in many areas of science. One amusing application is to the formation of cliques in social networks. Given a set of people, between any two people, assign a +1 for “like” or a –1 for “dislike.” Then the pattern of likes and dislikes has a coherence, internal consistency, or balance if they are arbitrage-free in the sense that the product around any circle is +1. An example that is not arbitrage-free, i.e., is unbalanced, is the famous mother-in-law triangle (the lines don’t need a direction in this case since +1 and –1 are equal to their own reciprocal).
The arbitrage-free law then gives the basic result about clique formation: given a set of people with either a “like” or “dislike” between some pairs, the set can be partitioned into two cliques (e.g., the Hatfields and McCoys, Montagues and Capulets, Serbs and Croatians, Sunnis and Shiites,…) so that all likes are intra-clique and all dislikes are inter-clique if and only if the pattern is balanced (i.e., the product around any circle is +1). In the balanced case, one can assign a +1 to one clique and a –1 to the other clique so that all the +1′s and –1′s on the lines between people are the ratios of the “prices.” When a pattern of likes and dislikes is unbalanced (i.e., not arbitrage-free), then instead of a good having two prices, some people, like the wife in the mother-in-law triangle (or Romeo and Juliet), in effect belong to two families or two cliques.
Gridlocked gears
The “graphical gridlock” referred to in the title of this posting refers to trains of intermeshing gears which are all in the same plane (e.g., could all be laid on a flat surface). If one turns one of the gears, that would in general transmit motion through the other gears. But suppose the gear train comes around in a circle? Then the possibility arises of the transmitted motion coming around in a circle to oppose the original motion so that the gear train would be rigid or gridlocked.
One usually has to pay attention to the number of teeth in each gear to calculate the transmitted motion since the revolutions per minute of the different gears would be determined by the gear ratios. But if all the gears were in the same plane, then each gear’s number of teeth would appear once in the numerator of a gear ratio and once in the denominator so they would always cancel out in absolute value. Thus all that counts is whether the gear is going clockwise or counter-clockwise.
In every gear meshing (not all gears have to mesh), the gears have to have different clockwise motions. If one is clockwise, the other is counter-clockwise and vice-versa. Thus each gear meshing is modeled as two people who “dislike” each other. All gear meshings are links with a –1. Then the arbitrage-free law has a very simple form since the product of –1′s is +1 if and only there are an even number of –1′s in the product. Hence we have:
a circular planar gear train can move if and only if there are an even number of gears in the circle.
In that arbitrage-free or balanced case, the two cliques are the “clockwise” gears and the “counter-clockwise” gears. Or putting the law the other way around,
a circular planar gear train has gridlock if and only if there are an odd number of gears.
When graphic artists try to represent some type of machinery in a logo or symbol, they seem drawn like moths to a flame to use a circular gear train of three gears all lying in a plane. But since 3 is a odd number, such a gear train is rigid as one can easily see by trying to assign the cliques “clockwise” and “counter-clockwise” to each gear in a consistent manner (so that each meshing will reverse the clockwise direction).
Examples of graphical gridlock
A good friend of mine is the head of a terrific group in Chicago dedicated to the rebirth of manufacturing jobs in the area and in America so it would be natural for them to have a logo with gears. To my surprise, the logo that first appeared on their newsletter was:
When I pointed out the “gridlock” implications, he said they would certainly change it as soon as they ran through the printing of their stationery.
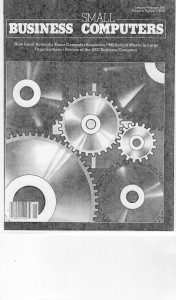
Over the years, I have collected examples of graphic artists picturing gridlock when they intend to picture smooth working machinery. For instance, The Economist magazine had the following cover to represent the “global factory.”
But one doubts they were trying to make the point that it does not work!
This global factory will not work.
Or a consulting company might compare their input as applying some oil to the company’s machinery.
This machinery needs more than oil.
Or taking a special training course may get one’s mental gears moving better, but not if they are in the following pattern.
This training course won’t help these gears.
Some graphic artists take a picture of real gears.
This gear train is only for the photograph.
Some graphic artists seem to finally get it when they put an even number of gears in a circular gear train. But then they are drawn again to the siren song of graphic gridlock by putting in the gear in the upper right corner that once again makes the whole gear train rigid.
The mathematical treatment of these ideas and the connection with optimization theory is downloadable here.
Addendum: May 3, 2010
My friend finally fixed his logo so it was no longer rigid.
