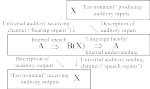Classical physics and quantum physics suggest two different meta-physical conceptions of reality: the classical notion of a objectively definite reality “all the way down,” and the quantum conception of an objectively indefinite type of reality. Part of the problem of interpreting quantum mechanics (QM) is the problem of making sense out of an objectively indefinite reality. Our sense-making strategy is to follow the math by showing that the mathematical way to describe indefiniteness is by partitions (quotient sets or equivalence relations).
Mac Lane, Bourbaki, and Adjoints Redux
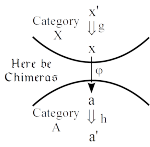
Saunders Mac Lane famously said that Bourbaki (in the person of Pierre Samuel) “just missed” developing the notion of an adjunction in 1948 a decade before Daniel Kan. When adjoints are defined using heteromorphisms or chimera morphisms (first done by Bodo Pareigis in 1970), then indeed Samuel was only a now-simple dualization away from defining adjoints. Since the heteromorphic treatment allows adjunctions to be factored into two, in general, independent universal mapping properties (UMPs), we also pose the question as to whether adjunctions or UMPs should be considered as category theory’s most important concept. It seems Mac Lane and most orthodox category theorists would say adjunctions but Grothendieck among others would say UMPs stated using representable functors.
On Adjoint and Brain Functors
We give a heterodox treatment of adjoints using heteromorphisms (object-to-object morphisms between objects of different categories) that parses an adjunction into two separate parts (left and right representations of heteromorphisms). Then these separate parts can be recombined in a new way to define a cognate concept, the brain functor, to abstractly model the functions of perception and action of a brain. This is a preprint of the paper coming out in Axiomathes.
Why Delayed Choice Experiments do Not imply Retrocausality
This is the on-line first version (unpaginated) of my first publication in quantum mechanics. It shows how delayed-choice experiments should be interpreted so they do not imply retrocausality.
Double-entry Bookkeeping: The Mathematical Treatment
This publication represents success in a long struggle, stretching over three decades, to get the mathematical treatment of double-entry bookkeeping published in an accounting journal.
Four Ways from Universal to Particular: How Chomsky is Not Selectionist
Chomsky’s mechanism (and embryonic development) are significantly different from the selectionist mechanisms of biological evolution or the immune system. Surprisingly, there is a very abstract way using two dual mathematical logics to make the distinction between genuinely selectionist mechanisms and what are better called “generative” mechanisms. This note outlines that distinction.
Partitions and Objective Indefiniteness in Quantum Mechanics
The problem of interpreting quantum mechanics (QM) is essentially the problem of making sense out of an objectively indefinite reality–that is described mathematically by partitions. Our sense-making strategy is implemented by developing the mathematics of partitions at the connected conceptual levels of sets and vector spaces. Set concepts are transported to (complex) vector spaces to yield the mathematical machinery of full QM, and the complex vector space concepts of full QM are transported to the set-like vector spaces over ℤ₂ to yield the rather fulsome pedagogical model of quantum mechanics over sets or QM/sets.
Introduction to Logical Entropy
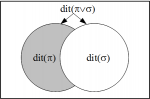
This paper, a reprint from the International Journal of Semantic Computing, introduces the logical notion of entropy based on the newly developed logic of partitions that is mathematically dual to the usual Boolean logic of subsets (aka “propositional logic”), and compares it to the usual Shannon entropy.
Quantum mechanics over sets
This paper gives a toy model of quantum mechanics over the field 2, where the vectors can be interpreted as subsets of a universe set, and hence the name: “Quantum mechanics over sets.” It gives the “logic” of QM in the old-fashioned sense of the essential logic of a theory pared down to operations on sets (vectors over 2). This includes the simplest logical treatment of the double-slit experiment, Bell’s Theorem, the probability calculus based on Born’s Rule, and much else (all restated in the context of sets).
Information as distinctions
This paper is sub-titled “New Foundations for Information Theory” since it is based on the logical notion of entropy from the logic of partitions. The basic logical idea is that of “distinctions.” Logical entropy is normalized counting measure of the set of distinctions of a partition, and Shannon entropy is the number of binary partitions needed, on average, to make the same distinctions of the partition.