The slogan “Follow the money” means that finding the source of an organization’s or person’s money may reveal their true nature. In a similar sense, we use the slogan “Follow the math!” to mean that finding “where the mathematics of QM comes from” reveals a good deal about the key concepts and machinery of the theory.
Partitions, Objective Indefiniteness, and Quantum Reality
December 30, 2022 by
This paper, published in the International Journal for Quantum Foundations, is a shorter introductory paper following up on my recent “Follow the Math!” paper in the Foundations of Physics. The point is to show that the mathematics of QM is the vector (Hilbert) space version of the mathematics of partitions at the set level. […]
Abstraction in Math and Superposition in QM
April 21, 2022 by
Abstraction turns equivalence into identity, but there are two ways to do it. The goal is to use the second notion of abstraction to shed some light on the notion of an inde finite superposition in quantum mechanics.
4Open: Special Issue: Intro. to Logical Entropy
January 14, 2022 by
4Open is a relatively new open access interdisciplinary journal (voluntary APCs) covering the 4 fields of mathematics, physics, chemistry, and biology-medicine. A special issue on Logical Entropy was sponsored and edited by Giovanni Manfredi, the Research Director of the CNRS Strasbourg. My paper is the introduction to the volume.
Book Draft: The Logic of Partitions
January 8, 2022 by
This book draft is an introduction to the logic of partitions on a set as well as the (quantum) logic of partitions (direct-sum decompositions or DSDs) on a vector space.
Book draft: Quantum Mechanics over Sets
January 8, 2022 by
Quantum mechanics overs sets (QM/ℤ₂ or QM/Sets) is a pedagogical or `toy’ model of finite-dimensional quantum mechanics (QM/ℂ) that reproduces in the simplified setting of vector spaces over ℤ₂ the essentials of projective measurements, the double-slit experiment, the indeterminacy principle, entanglement, Bell’s Theorem, the statistics of indistinguishable particles, and so forth,
The Logical Theory of Canonical Maps
July 11, 2020 by
The purpose of this paper is to show that the dual notions of elements & distinctions are the basic analytical concepts needed to unpack and analyze morphisms, duality, and universal constructions in the Sets, the category of sets and functions.
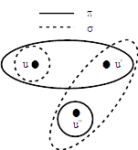
Negation and Implication in Partition Logic
July 4, 2020 by
Our purpose in this paper is to explore the notions of negation and implication in that other mathematical logic of partitions.
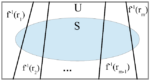
Probability Theory with Superposition Events
June 18, 2020 by
In finite probability theory, events are subsets S⊆U of the outcome set. Subsets can be represented by 1-dimensional column vectors. By extending the representation of events to two dimensional matrices, we can introduce “superposition events.”
Extending All Boolean Operations to Partitions
June 11, 2019 by
The lattice operations of join and meet were defined for set partitions in the nineteenth century, but no new logical operations on partitions were defined and studied during the twentieth century. Yet there is a simple and natural graph-theoretic method presented here to define any n-ary Boolean operation on partitions. An equivalent closure-theoretic method is […]