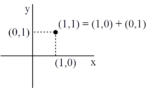This paper tries to elucidate the paradoxical aspects of quantum mechanics (QM) by using a simplified pedagogical model of QM based on the support sets of the state vectors, by assuming an ontology of superposition-as-objective indefiniteness, and by not giving any ontological interpretation to the computational device of the wave function.
Book draft: Quantum Mechanics over Sets
January 8, 2022 by
Quantum mechanics overs sets (QM/ℤ₂ or QM/Sets) is a pedagogical or `toy’ model of finite-dimensional quantum mechanics (QM/ℂ) that reproduces in the simplified setting of vector spaces over ℤ₂ the essentials of projective measurements, the double-slit experiment, the indeterminacy principle, entanglement, Bell’s Theorem, the statistics of indistinguishable particles, and so forth,
Probability Theory with Superposition Events
June 18, 2020 by
In finite probability theory, events are subsets S⊆U of the outcome set. Subsets can be represented by 1-dimensional column vectors. By extending the representation of events to two dimensional matrices, we can introduce “superposition events.”
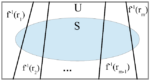
Quantum Logic of Direct-sum Decompositions
June 20, 2017 by
The usual quantum logic, beginning with Birkhoff and Von Neumann, was the logic of closed subspaces of a Hilbert space. This paper develops the more general logic of direct-sum decompositions of a vector space. This allows the treatment of measurement of any self-adjoint operators rather than just the projection operators associated with subspaces.
On Classical and Quantum Logical Entropy: The analysis of measurement
September 8, 2016 by
This paper shows how classical and quantum logical entropy arise out of the logic of partitions, and then it shows how there is a natural connection between the nxn distinctions and indistinctions of a partition and the nxn entries in a density matrix so that the classical and quantum logical entropy can directly register what happens to the density matrix in a projective measurement. The standard notion of von Neumann entropy does nothing of the kind–so the paper is also an indirect critique of von Neumann entropy as the most natural and ‘informative’ notion of entropy to use in quantum information theory.
Quantum Mechanics over Sets
August 8, 2016 by
This paper published in Synthese shows how the classical finite probability theory (with equiprobable outcomes) can be reinterpreted and recast as the quantum probability calculus of a pedagogical or “toy” model of quantum mechanics over sets (QM/sets).