This is a set of slides from a talk on introducing the Hamming distance into classical logical information theory and then developing the quantum logical notion of Hamming distance–which turns out to equal a standard notion of distance in quantum information theory, the Hilbert-Schmidt distance.
Talk: New Foundations for Quantum Information Theory
November 28, 2017 by
These are the slides for a talk given at the 6th International Conference on New Frontiers in Physics on Crete in August 2017.
Logical Entropy: Introduction to Classical and Quantum Logical Information Theory
July 15, 2017 by
Logical information theory is the quantitative version of the logic of partitions just as logical probability theory is the quantitative version of the dual Boolean logic of subsets. The resulting notion of information is about distinctions, differences, and distinguishability, and is formalized as the distinctions of a partition (a pair of points distinguished by the partition). This paper is an introduction to the quantum version of logical information theory.
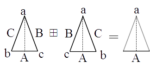
Quantum Logic of Direct-sum Decompositions
June 20, 2017 by
The usual quantum logic, beginning with Birkhoff and Von Neumann, was the logic of closed subspaces of a Hilbert space. This paper develops the more general logic of direct-sum decompositions of a vector space. This allows the treatment of measurement of any self-adjoint operators rather than just the projection operators associated with subspaces.
From Abstraction in Math to Superposition in QM
January 14, 2017 by
This is a draft paper that makes a perhaps surprising connection between the old Platonic notion of a paradigm-universal like ‘the white thing’ and an indefinite superposition state in quantum mechanics.
Quantum Mechanics over Sets
August 8, 2016 by
This paper published in Synthese shows how the classical finite probability theory (with equiprobable outcomes) can be reinterpreted and recast as the quantum probability calculus of a pedagogical or “toy” model of quantum mechanics over sets (QM/sets).
The Existence-Information Duality
October 30, 2015 by
The development of the logic of partitions (dual to the usual Boolean logic of subsets) and logical information theory bring out a fundamental duality between existence (e.g., elements of a subset) and information (e.g., distinctions of a partition). This leads in a more meta-physical vein to two different conceptions of reality, one of which provides the realistic interpretation of quantum mechanics.
Partition Logic talk slides Ljubljana
September 23, 2015 by
Slides from talk on Partition Logic at University of Ljubljana Sept. 8, 2015.
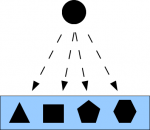
On the Objective Indefiniteness Interpretation of Quantum Mechanics
August 28, 2015 by
Classical physics and quantum physics suggest two different meta-physical conceptions of reality: the classical notion of a objectively definite reality “all the way down,” and the quantum conception of an objectively indefinite type of reality. Part of the problem of interpreting quantum mechanics (QM) is the problem of making sense out of an objectively indefinite reality. Our sense-making strategy is to follow the math by showing that the mathematical way to describe indefiniteness is by partitions (quotient sets or equivalence relations).
Why Delayed Choice Experiments do Not imply Retrocausality
January 23, 2015 by
This is the on-line first version (unpaginated) of my first publication in quantum mechanics. It shows how delayed-choice experiments should be interpreted so they do not imply retrocausality.